Guys, I have tried to distill this information down to something the technically minded guitarist can understand. However, one may have to read through the post a couple of times.
There is a lot myth surrounding tube amps. That is mainly because myth is easier for the layman to understand than science. One of the main myths is tube watts are louder than solid-state watts. That is complete nonsense, a watt is a watt. A watt is equivalent to one joule of energy per second. There are couple of reasons for this myth. A big reason is that solid-state amp designers were not always honest about power ratings, preferring to state power in peak power terms instead of RMS power, which led to inflated power ratings.
That being said, the reason why tube amp often sounds louder than solid-state amps of the same RMS rating is because tube amps are poorly damped. Damping is the ratio between speaker impedance and amplifier output impedance. Damping factor determines how well the power stage of an amp can control speaker cone movement. Power tubes have high output impedances. In order to drive a speaker, they require an output transformer that steps the power tube output impedance down to the speaker nominal impedance. In the process, the output transformer converts a high voltage, low current signal to a lower voltage, higher current signal. It also increases that amp's damping factor somewhat. For example, A Fender Vibrolux has an output transformer with a primary impedance of 4,000 (4K) ohms with secondary impedance of 4 ohms. However, an output transformer knows nothing about impedances. It works using impedance ratios, that is, if we plug an 8-ohm speaker into the 4-ohm jack, the power tubes will see primary impedance of 8,000 ohms instead of 4,000 ohms because any change in the load attached to the output transformer's secondary winding (a.k.a. the wind that is attached to the speaker jack) is reflected back to the power tubes. This change is due the fact that output transformers actually have impedance ratios, not fixed impedances. The impedance ratio is the ratio between the primary and secondary impedances. In this case, the impedance ratio is 4000 / 4 = 1000. The impedance ratio is the square of the ratio between the number of turns of wire in the primary and the secondary windings, which is SQRT(1000) ~= 32, where SQRT is the square-root function. What that means in layman’s terms is that there is one turn of wire in the secondary winding for every thirty-two turns in the primary winding.
Now, lets get to the meat of why tubes amp tend to sound lively and whereas solid-state designs often sound flat. That is because impedance is not a synonym for DC resistance. Impedance is an AC measurement that contains reactive components; namely, inductive reactance and capacitive reactance. The formula for computing impedance is:
Z = SQRT(R^2 + (Xl – Xc)^2), where SQRT is the square-root formula, R = DC resistance, Xl = inductive reactance, and Xc = capacitive reactance.
Xl = 2 * 3.14 * f * L, where f = frequency, L = inductance henries
Xc = 1 / (2 * 3.14 * f * C), where f = frequency, C = capacitance in farads
The important takeaway here is that both Xl and Xc change with respect to frequency, which means that impedances changes with respect to frequency. DC resistance does not change with respect to frequency, which is why impedance is not a synonym for DC resistance.
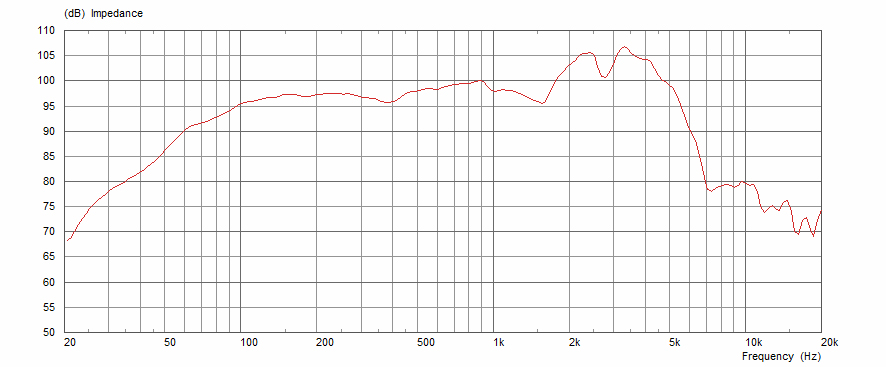
Anyone who has ever measured the DC resistance of a speaker knows that is usually not equal to a speaker’s rated impedance. That is because a speaker’s impedance rating is based on the average of its lowest impedance values. An ohm meter uses DC current for measurement, which is zero hertz; therefore, we are measuring the resistance of speaker with the equivalent a zero hertz signal.
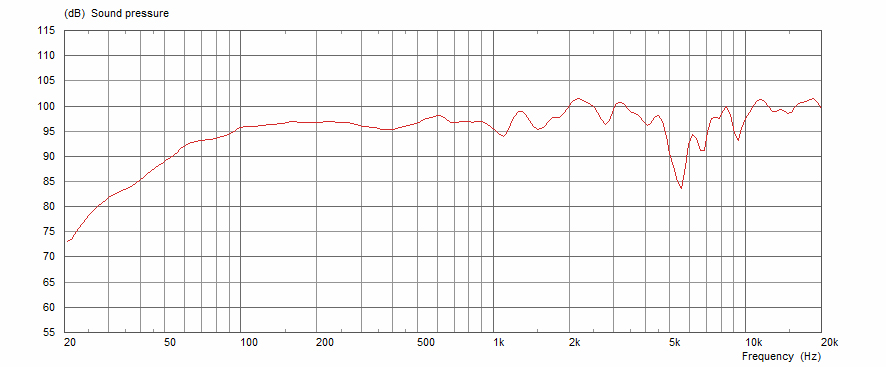
Now, the we know that impedance changes with respect to frequency and an output transformer is merely a set of impedance ratios in which the primary impedance is based on the impedance of the load, we can move on to why tube amps tend to sound more lively and louder than most solid-state designs. That is because the output of a tube amp is a constant current source and voltage (E) equals current (I) times resistance (R). However, in our case, impedance (Z) is substituted for resistance. Now, we are in a position to understand why a tube amp often sounds more lively and louder than the average solid-state amp. The impedance of a speaker generally increases with respect to frequency, which causes the voltage applied to the speaker to increase with frequency because voltage equals current times impedance. This change causes an increase in power to be delivered to the load because the current remains constant and power in watts (w) is equal to voltage times current. This effect is unwanted in hi-fi because hi-fi seeks to have even frequency response. Poor damping is the reason why all but the most expensive tube audio power amps have less detail than the average solid-state power amp. In essence, poor damping is a technological foible that guitarists exploited to their advantage.
Most of the second generation of solid-state music amp designs are highly damped because the amplifier’s output impedance is lower than the speaker impedance. High damping factor allows these amps to have much tighter control over speaker cone movement, which is why bass guitarists embraced solid-state amps. A bass is tuned an octave lower than a guitar and low notes require much larger cone movement than high notes, so a high damping factor is boon to bass guitar. Second generation solid-state amps also operate as constant voltage sources instead of constant current sources. That is why power delivered to the load increases when output impedance decreases. If we hold voltage steady and half the impedance of the load we double the amount of current that is flowing through the load because current (I) is equal to voltage (E) divided by impedance (Z). This way of reacting to speaker load is the exact opposite of what happens in a tube amp, meaning that the current delivered to the load decreases with respect to frequency because impedance increases with respect to frequency. If current delivered to the load decreases, power delivered to the load decreases, which results in higher notes not popping like they do with a tube amp.
Now, there is a way to reduce damping factor and make a solid-state power stage behave much like a tube output stage. That is done by changing the topology such that the speaker forms part of the resistive divider that is the feedback loop. The resistance used to ground, Rg, of the resistive divider used for the feedback loop is less than the nominal impedance of the speaker, which functions as Rf. The feedback loop determines the gain of the output stage. The formula for gain equals Rf divided by Rg plus 1. If Rf goes up, the gain of the circuit goes up; hence, it causes power to increase with increases in load impedance much like a tube amp. Marshall first used this topology with their ValveState amps. The tube in the preamp is about as much for show as it is for sound.
There is a lot myth surrounding tube amps. That is mainly because myth is easier for the layman to understand than science. One of the main myths is tube watts are louder than solid-state watts. That is complete nonsense, a watt is a watt. A watt is equivalent to one joule of energy per second. There are couple of reasons for this myth. A big reason is that solid-state amp designers were not always honest about power ratings, preferring to state power in peak power terms instead of RMS power, which led to inflated power ratings.
That being said, the reason why tube amp often sounds louder than solid-state amps of the same RMS rating is because tube amps are poorly damped. Damping is the ratio between speaker impedance and amplifier output impedance. Damping factor determines how well the power stage of an amp can control speaker cone movement. Power tubes have high output impedances. In order to drive a speaker, they require an output transformer that steps the power tube output impedance down to the speaker nominal impedance. In the process, the output transformer converts a high voltage, low current signal to a lower voltage, higher current signal. It also increases that amp's damping factor somewhat. For example, A Fender Vibrolux has an output transformer with a primary impedance of 4,000 (4K) ohms with secondary impedance of 4 ohms. However, an output transformer knows nothing about impedances. It works using impedance ratios, that is, if we plug an 8-ohm speaker into the 4-ohm jack, the power tubes will see primary impedance of 8,000 ohms instead of 4,000 ohms because any change in the load attached to the output transformer's secondary winding (a.k.a. the wind that is attached to the speaker jack) is reflected back to the power tubes. This change is due the fact that output transformers actually have impedance ratios, not fixed impedances. The impedance ratio is the ratio between the primary and secondary impedances. In this case, the impedance ratio is 4000 / 4 = 1000. The impedance ratio is the square of the ratio between the number of turns of wire in the primary and the secondary windings, which is SQRT(1000) ~= 32, where SQRT is the square-root function. What that means in layman’s terms is that there is one turn of wire in the secondary winding for every thirty-two turns in the primary winding.
Now, lets get to the meat of why tubes amp tend to sound lively and whereas solid-state designs often sound flat. That is because impedance is not a synonym for DC resistance. Impedance is an AC measurement that contains reactive components; namely, inductive reactance and capacitive reactance. The formula for computing impedance is:
Z = SQRT(R^2 + (Xl – Xc)^2), where SQRT is the square-root formula, R = DC resistance, Xl = inductive reactance, and Xc = capacitive reactance.
Xl = 2 * 3.14 * f * L, where f = frequency, L = inductance henries
Xc = 1 / (2 * 3.14 * f * C), where f = frequency, C = capacitance in farads
The important takeaway here is that both Xl and Xc change with respect to frequency, which means that impedances changes with respect to frequency. DC resistance does not change with respect to frequency, which is why impedance is not a synonym for DC resistance.
Anyone who has ever measured the DC resistance of a speaker knows that is usually not equal to a speaker’s rated impedance. That is because a speaker’s impedance rating is based on the average of its lowest impedance values. An ohm meter uses DC current for measurement, which is zero hertz; therefore, we are measuring the resistance of speaker with the equivalent a zero hertz signal.
Now, the we know that impedance changes with respect to frequency and an output transformer is merely a set of impedance ratios in which the primary impedance is based on the impedance of the load, we can move on to why tube amps tend to sound more lively and louder than most solid-state designs. That is because the output of a tube amp is a constant current source and voltage (E) equals current (I) times resistance (R). However, in our case, impedance (Z) is substituted for resistance. Now, we are in a position to understand why a tube amp often sounds more lively and louder than the average solid-state amp. The impedance of a speaker generally increases with respect to frequency, which causes the voltage applied to the speaker to increase with frequency because voltage equals current times impedance. This change causes an increase in power to be delivered to the load because the current remains constant and power in watts (w) is equal to voltage times current. This effect is unwanted in hi-fi because hi-fi seeks to have even frequency response. Poor damping is the reason why all but the most expensive tube audio power amps have less detail than the average solid-state power amp. In essence, poor damping is a technological foible that guitarists exploited to their advantage.
Most of the second generation of solid-state music amp designs are highly damped because the amplifier’s output impedance is lower than the speaker impedance. High damping factor allows these amps to have much tighter control over speaker cone movement, which is why bass guitarists embraced solid-state amps. A bass is tuned an octave lower than a guitar and low notes require much larger cone movement than high notes, so a high damping factor is boon to bass guitar. Second generation solid-state amps also operate as constant voltage sources instead of constant current sources. That is why power delivered to the load increases when output impedance decreases. If we hold voltage steady and half the impedance of the load we double the amount of current that is flowing through the load because current (I) is equal to voltage (E) divided by impedance (Z). This way of reacting to speaker load is the exact opposite of what happens in a tube amp, meaning that the current delivered to the load decreases with respect to frequency because impedance increases with respect to frequency. If current delivered to the load decreases, power delivered to the load decreases, which results in higher notes not popping like they do with a tube amp.
Now, there is a way to reduce damping factor and make a solid-state power stage behave much like a tube output stage. That is done by changing the topology such that the speaker forms part of the resistive divider that is the feedback loop. The resistance used to ground, Rg, of the resistive divider used for the feedback loop is less than the nominal impedance of the speaker, which functions as Rf. The feedback loop determines the gain of the output stage. The formula for gain equals Rf divided by Rg plus 1. If Rf goes up, the gain of the circuit goes up; hence, it causes power to increase with increases in load impedance much like a tube amp. Marshall first used this topology with their ValveState amps. The tube in the preamp is about as much for show as it is for sound.
Last edited: